>>> from numpy import * >>> import operator >>> a = array([[1,2,3],[4,5,6]]) >>> a array([[1, 2, 3], [4, 5, 6]]) >>> m = mat(a) >>> m matrix([[1, 2, 3], [4, 5, 6]]) >>> a ** 2 array([[ 1, 4, 9], [16, 25, 36]]) >>> m ** 2 Traceback (most recent call last): File "<stdin>", line 1, in <module> File "D:\anaconda\lib\site-packages\numpy\matrixlib\defmatrix.py", line 356, in __pow__ return matrix_power(self, other) File "D:\anaconda\lib\site-packages\numpy\matrixlib\defmatrix.py", line 173, in matrix_power raise ValueError("input must be a square array") ValueError: input must be a square array >>> 

>>> A = array([[1,2,3],[4,5,6],[7,8,9]]) >>> A array([[1, 2, 3], [4, 5, 6], [7, 8, 9]]) >>> M = mat(A) >>> M matrix([[1, 2, 3], [4, 5, 6], [7, 8, 9]]) >>> A ** 2 array([[ 1, 4, 9], [16, 25, 36], [49, 64, 81]]) >>> M ** 2 matrix([[ 30, 36, 42], [ 66, 81, 96], [102, 126, 150]])

本文分享自微信公众号 - 福大大架构师每日一题（gh_bbe96e5def84）。
如有侵权，请联系 support@oschina.cn 删除。
本文参与 “OSC 源创计划”，欢迎正在阅读的你也加入，一起分享。

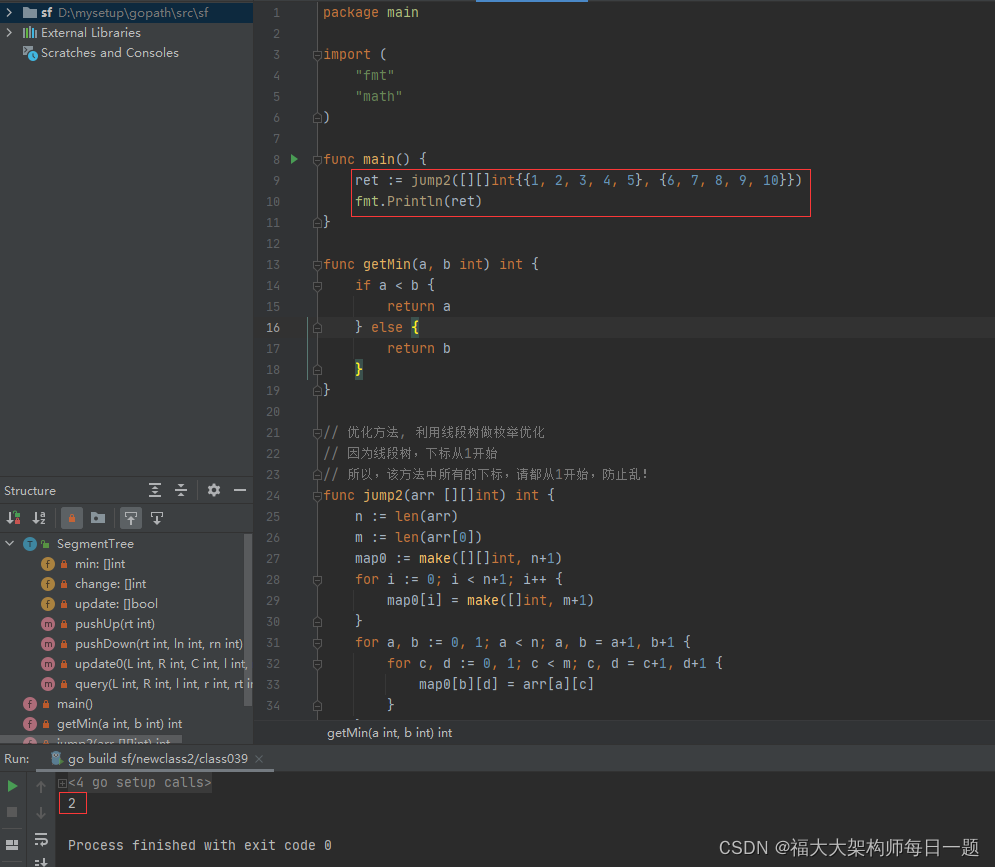
2021-12-28：给定一个二维数组 matrix，matrix [i][j] = k 代表：从 (i,j) 位置可以随意往右跳＜=k 步，或者从 (i,j) 位置可以随意往下跳＜=k 步， 如果 matrix [i]

2021-12-28：给定一个二维数组 matrix，matrix [i][j] = k 代表:
从 (i,j) 位置可以随意往右跳 <=k 步，或者从 (i,j) 位置可以随意往下跳 <=k 步，
如果 matrix [i][j] = 0，代表来到 (i,j) 位置必须停止，
返回从 matrix 左上角到右下角，至少要跳几次，
已知 matrix 中行数 n <= 5000, 列数 m <= 5000，
matrix 中的值，<= 5000。
来自京东。

答案 2021-12-28：

方法一：自然智慧。递归。复杂度过不了。
方法二：动态规划 + 线段树。

代码用 golang 编写。代码如下：

package main

import (
    "fmt"
    "math"
)

func main() {
   
    ret := jump2([][]int{
   {
   1, 2, 3, 4, 5}, {
   6, 7, 8, 9, 10}})
    fmt.Println(ret)
}

func getMin(a, b int) int {
   
    if a < b {
   
        return a
    } else {
   
        return b
    }
}

// 优化方法, 利用线段树做枚举优化
// 因为线段树，下标从1开始
// 所以，该方法中所有的下标，请都从1开始，防止乱！
func jump2(arr [][]int) int {
   
    n := len(arr)
    m := len(arr[0])
    map0 := make([][]int, n+1)
    for i := 0; i < n+1; i++ {
   
        map0[i] = make([]int, m+1)
    }
    for a, b := 0, 1; a < n; a, b = a+1, b+1 {
   
        for c, d := 0, 1; c < m; c, d = c+1, d+1 {
   
            map0[b][d] = arr[a][c]
        }
    }
    rowTrees := make([]*SegmentTree, n+1)
    for i := 1; i <= n; i++ {
   
        rowTrees[i] = NewSegmentTree(m)
    }
    colTrees := make([]*SegmentTree, m+1)
    for i := 1; i <= m; i++ {
   
        colTrees[i] = NewSegmentTree(n)
    }
    rowTrees[n].update0(m, m, 0, 1, m, 1)
    colTrees[m].update0(n, n, 0, 1, n, 1)
    for col := m - 1; col >= 1; col-- {
   
        if map0[n][col] != 0 {
   
            left := col + 1
            right := getMin(col+map0[n][col], m)
            next := rowTrees[n].query(left, right, 1, m, 1)
            if next != math.MaxInt64 {
   
                rowTrees[n].update0(col, col, next+1, 1, m, 1)
                colTrees[col].update0(n, n, next+1, 1, n, 1)
            }
        }
    }
    for row := n - 1; row >= 1; row-- {
   
        if map0[row][m] != 0 {
   
            up := row + 1
            down := getMin(row+map0[row][m], n)
            next := colTrees[m].query(up, down, 1, n, 1)
            if next != math.MaxInt64 {
   
                rowTrees[row].update0(m, m, next+1, 1, m, 1)
                colTrees[m].update0(row, row, next+1, 1, n, 1)
            }
        }
    }
    for row := n - 1; row >= 1; row-- {
   
        for col := m - 1; col >= 1; col-- {
   
            if map0[row][col] != 0 {
   
                // (row,col) 往右是什么范围呢？[left,right]
                left := col + 1
                right := getMin(col+map0[row][col], m)
                next1 := rowTrees[row].query(left, right, 1, m, 1)
                // (row,col) 往下是什么范围呢？[up,down]
                up := row + 1
                down := getMin(row+map0[row][col], n)
                next2 := colTrees[col].query(up, down, 1, n, 1)
                next := getMin(next1, next2)
                if next != math.MaxInt64 {
   
                    rowTrees[row].update0(col, col, next+1, 1, m, 1)
                    colTrees[col].update0(row, row, next+1, 1, n, 1)
                }
            }
        }
    }
    return rowTrees[1].query(1, 1, 1, m, 1)
}

// 区间查询最小值的线段树
// 注意下标从1开始，不从0开始
// 比如你传入size = 8
// 则位置对应为1~8，而不是0~7
type SegmentTree struct {
   
    min    []int
    change []int
    update []bool
}

func NewSegmentTree(size int) *SegmentTree {
   
    ret := &SegmentTree{
   }
    N := size + 1
    ret.min = make([]int, N<<2)
    ret.change = make([]int, N<<2)
    ret.update = make([]bool, N<<2)
    ret.update0(1, size, math.MaxInt64, 1, size, 1)
    return ret
}

func (this *SegmentTree) pushUp(rt int) {
   
    this.min[rt] = getMin(this.min[rt<<1], this.min[rt<<1|1])
}

func (this *SegmentTree) pushDown(rt, ln, rn int) {
   
    if this.update[rt] {
   
        this.update[rt<<1] = true
        this.update[rt<<1|1] = true
        this.change[rt<<1] = this.change[rt]
        this.change[rt<<1|1] = this.change[rt]
        this.min[rt<<1] = this.change[rt]
        this.min[rt<<1|1] = this.change[rt]
        this.update[rt] = false
    }
}

// 最后三个参数是固定的, 每次传入相同的值即可:
// l = 1(固定)
// r = size(你设置的线段树大小)
// rt = 1(固定)
func (this *SegmentTree) update0(L, R, C, l, r, rt int) {
   
    if L <= l && r <= R {
   
        this.update[rt] = true
        this.change[rt] = C
        this.min[rt] = C
        return
    }
    mid := (l + r) >> 1
    this.pushDown(rt, mid-l+1, r-mid)
    if L <= mid {
   
        this.update0(L, R, C, l, mid, rt<<1)
    }
    if R > mid {
   
        this.update0(L, R, C, mid+1, r, rt<<1|1)
    }
    this.pushUp(rt)
}

// 最后三个参数是固定的, 每次传入相同的值即可:
// l = 1(固定)
// r = size(你设置的线段树大小)
// rt = 1(固定)
func (this *SegmentTree) query(L, R, l, r, rt int) int {
   
    if L <= l && r <= R {
   
        return this.min[rt]
    }
    mid := (l + r) >> 1
    this.pushDown(rt, mid-l+1, r-mid)
    left := math.MaxInt64
    right := math.MaxInt64
    if L <= mid {
   
        left = this.query(L, R, l, mid, rt<<1)
    }
    if R > mid {
   
        right = this.query(L, R, mid+1, r, rt<<1|1)
    }
    return getMin(left, right)
}

执行结果如下：

左神 java 代码

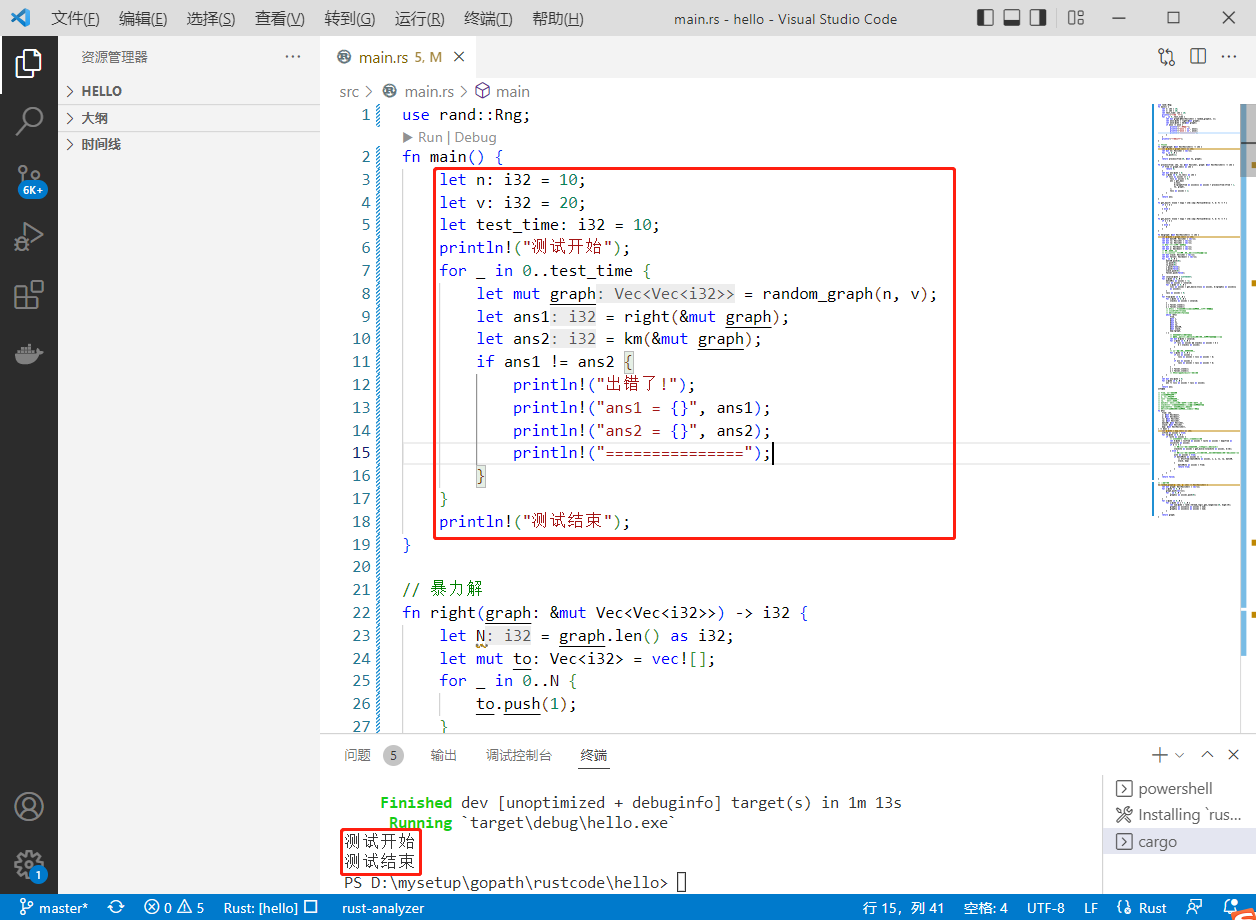
2022-06-11：注意本文件中，graph 不是邻接矩阵的含义，而是一个二部图。 在长度为 N 的邻接矩阵 matrix 中，所有的点有 N 个，matrix [i][j] 表示点 i 到点 j 的距离或者权重， 而在二部

2022-06-11：注意本文件中，graph 不是邻接矩阵的含义，而是一个二部图。 在长度为 N 的邻接矩阵 matrix 中，所有的点有 N 个，matrix [i][j] 表示点 i 到点 j 的距离或者权重， 而在二部图 graph 中，所有的点有 2*N 个，行所对应的点有 N 个，列所对应的点有 N 个。 而且认为，行所对应的点之间是没有路径的，列所对应的点之间也是没有路径的！

答案 2022-06-11：

km 算法。

代码用 rust 编写。代码如下：

use rand::Rng;
fn main() {
    let n: i32 = 10;
    let v: i32 = 20;
    let test_time: i32 = 10;
    println!("测试开始");
    for _ in 0..test_time {
        let mut graph = random_graph(n, v);
        let ans1 = right(&mut graph);
        let ans2 = km(&mut graph);
        if ans1 != ans2 {
            println!("出错了!");
            println!("ans1 = {}", ans1);
            println!("ans2 = {}", ans2);
            println!("===============");
        }
    }
    println!("测试结束");
}

// 暴力解
fn right(graph: &mut Vec<Vec<i32>>) -> i32 {
    let N = graph.len() as i32;
    let mut to: Vec<i32> = vec![];
    for _ in 0..N {
        to.push(1);
    }
    return process(0, &mut to, graph);
}

fn process(from: i32, to: &mut Vec<i32>, graph: &mut Vec<Vec<i32>>) -> i32 {
    if from == graph.len() as i32 {
        return 0;
    }
    let mut ans = 0;
    for i in 0..to.len() as i32 {
        if to[i as usize] == 1 {
            to[i as usize] = 0;
            ans = get_max(
                ans,
                graph[from as usize][i as usize] + process(from + 1, to, graph),
            );
            to[i as usize] = 1;
        }
    }
    return ans;
}

fn get_max<T: Clone + Copy + std::cmp::PartialOrd>(a: T, b: T) -> T {
    if a > b {
        a
    } else {
        b
    }
}

fn get_min<T: Clone + Copy + std::cmp::PartialOrd>(a: T, b: T) -> T {
    if a < b {
        a
    } else {
        b
    }
}

fn km(graph: &mut Vec<Vec<i32>>) -> i32 {
    let N = graph.len() as i32;
    let mut match0: Vec<i32> = vec![];
    let mut lx: Vec<i32> = vec![];
    let mut ly: Vec<i32> = vec![];
    // dfs过程中，碰过的点！
    let mut x: Vec<bool> = vec![];
    let mut y: Vec<bool> = vec![];
    // 降低的预期！
    // 公主上，打一个，降低预期的值，只维持最小！
    let mut slack: Vec<i32> = vec![];
    let mut falsev: Vec<bool> = vec![];
    for _ in 0..N {
        match0.push(0);
        lx.push(0);
        ly.push(0);
        x.push(false);
        y.push(false);
        slack.push(0);
        falsev.push(false);
    }
    let invalid = 2147483647;
    for i in 0..N {
        match0[i as usize] = -1;
        lx[i as usize] = -invalid;
        for j in 0..N {
            lx[i as usize] = get_max(lx[i as usize], graph[i as usize][j as usize]);
        }
        ly[i as usize] = 0;
    }
    for from in 0..N {
        for i in 0..N {
            slack[i as usize] = invalid;
        }
        x = falsev.clone();
        y = falsev.clone();
        // dfs() : from王子，能不能不降预期，匹配成功！
        // 能：dfs返回true！
        // 不能：dfs返回false！
        while !dfs(
            from,
            &mut x,
            &mut y,
            &mut lx,
            &mut ly,
            &mut match0,
            &mut slack,
            graph,
        ) {
            // 刚才的dfs，失败了！
            // 需要拿到，公主的slack里面，预期下降幅度的最小值！
            let mut d = invalid;
            for i in 0..N {
                if !y[i as usize] && slack[i as usize] < d {
                    d = slack[i as usize];
                }
            }
            // 按照最小预期来调整预期
            for i in 0..N {
                if x[i as usize] {
                    lx[i as usize] = lx[i as usize] - d;
                }
                if y[i as usize] {
                    ly[i as usize] = ly[i as usize] + d;
                }
            }
            x = falsev.clone();
            y = falsev.clone();
            // 然后回到while里，再次尝试
        }
    }
    let mut ans = 0;
    for i in 0..N {
        ans += lx[i as usize] + ly[i as usize];
    }
    return ans;
}

// from, 当前的王子
// x，王子碰没碰过
// y, 公主碰没碰过
// lx，所有王子的预期
// ly, 所有公主的预期
// match，所有公主，之前的分配，之前的爷们！
// slack，连过，但没允许的公主，最小下降的幅度
// map，报价，所有王子对公主的报价
// 返回，from号王子，不降预期能不能配成！
fn dfs(
    from: i32,
    x: &mut Vec<bool>,
    y: &mut Vec<bool>,
    lx: &mut Vec<i32>,
    ly: &mut Vec<i32>,
    match0: &mut Vec<i32>,
    slack: &mut Vec<i32>,
    map: &mut Vec<Vec<i32>>,
) -> bool {
    let N = map.len() as i32;
    x[from as usize] = true;
    for to in 0..N {
        if !y[to as usize] {
            // 只有没dfs过的公主，才会去尝试
            let d = lx[from as usize] + ly[to as usize] - map[from as usize][to as usize];
            if d != 0 {
                // 如果当前的路不符合预期，更新公主的slack值
                slack[to as usize] = get_min(slack[to as usize], d);
            } else {
                // 如果当前的路符合预期，尝试直接拿下，或者抢夺让之前的安排倒腾去
                y[to as usize] = true;
                if match0[to as usize] == -1
                    || dfs(match0[to as usize], x, y, lx, ly, match0, slack, map)
                {
                    match0[to as usize] = from;
                    return true;
                }
            }
        }
    }
    return false;
}

// 为了测试
fn random_graph(N: i32, V: i32) -> Vec<Vec<i32>> {
    let mut graph: Vec<Vec<i32>> = vec![];
    for i in 0..N {
        graph.push(vec![]);
        for _ in 0..N {
            graph[i as usize].push(0);
        }
    }
    for i in 0..N {
        for j in i + 1..N {
            let num = rand::thread_rng().gen_range(0, V);
            graph[i as usize][j as usize] = num;
            graph[j as usize][i as usize] = num;
        }
    }
    return graph;
}

执行结果如下：

左神 java 代码

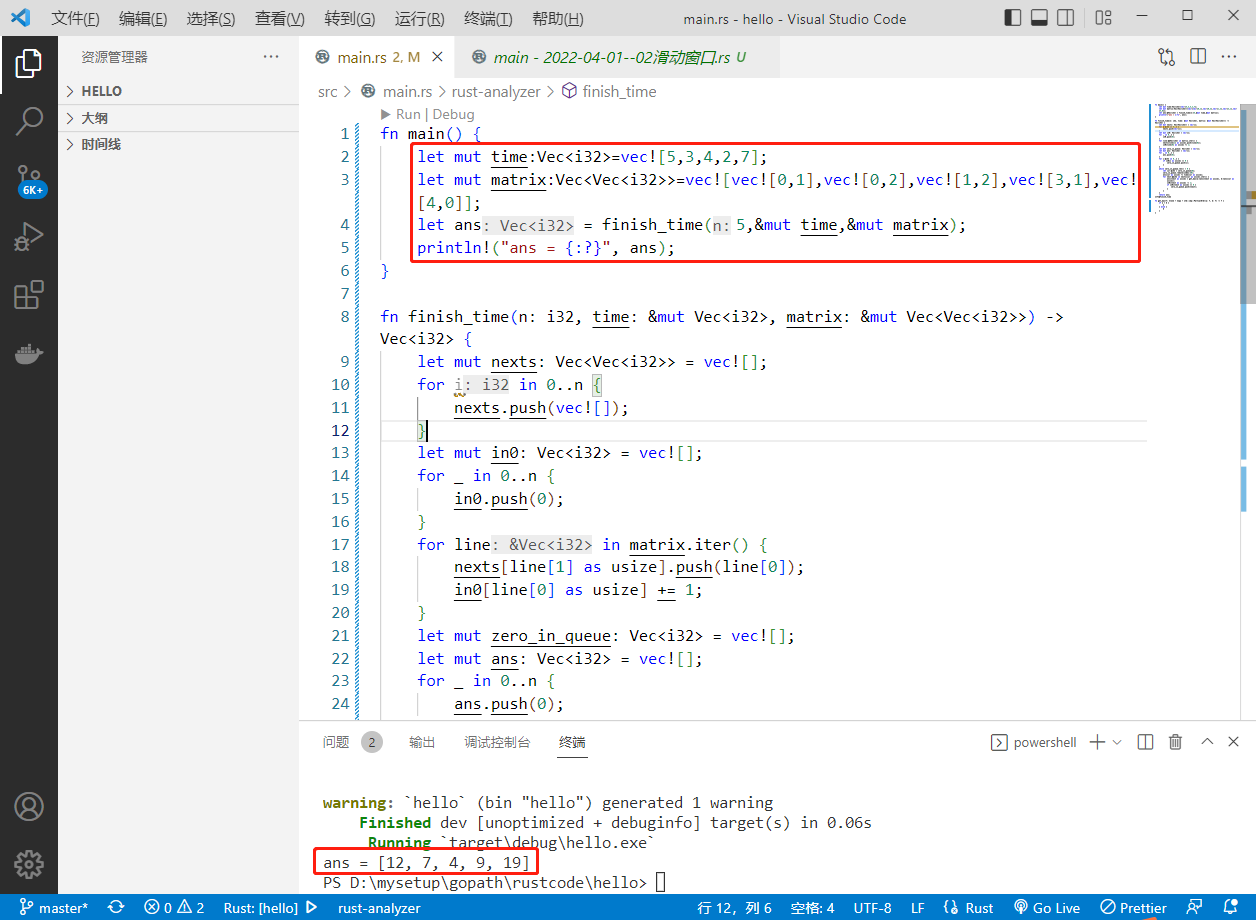
2022-06-25：给定一个正数 n, 表示有 0~n-1 号任务， 给定一个长度为 n 的数组 time，time [i] 表示 i 号任务做完的时间， 给定一个二维数组 matrix， matrix [j] = {a,

2022-06-25：给定一个正数 n, 表示有 0~n-1 号任务， 给定一个长度为 n 的数组 time，time [i] 表示 i 号任务做完的时间， 给定一个二维数组 matrix， matrix [j] = {a, b} 代表：a 任务想要开始，依赖 b 任务的完成， 只要能并行的任务都可以并行，但是任何任务只有依赖的任务完成，才能开始。 返回一个长度为 n 的数组 ans，表示每个任务完成的时间。 输入可以保证没有循环依赖。 来自美团。3.26 笔试。

答案 2022-06-25：

拓扑排序基础上做动态规划。

代码用 rust 编写。代码如下：

fn main() {
    let mut time:Vec<i32>=vec![5,3,4,2,7];
    let mut matrix:Vec<Vec<i32>>=vec![vec![0,1],vec![0,2],vec![1,2],vec![3,1],vec![4,0]];
    let ans = finish_time(5,&mut time,&mut matrix);
    println!("ans = {:?}", ans);
}

fn finish_time(n: i32, time: &mut Vec<i32>, matrix: &mut Vec<Vec<i32>>) -> Vec<i32> {
    let mut nexts: Vec<Vec<i32>> = vec![];
    for i in 0..n {
        nexts.push(vec![]);
    }
    let mut in0: Vec<i32> = vec![];
    for _ in 0..n {
        in0.push(0);
    }
    for line in matrix.iter() {
        nexts[line[1] as usize].push(line[0]);
        in0[line[0] as usize] += 1;
    }
    let mut zero_in_queue: Vec<i32> = vec![];
    let mut ans: Vec<i32> = vec![];
    for _ in 0..n {
        ans.push(0);
    }
    for i in 0..n {
        if in0[i as usize] == 0 {
            zero_in_queue.push(i);
        }
    }
    while zero_in_queue.len() > 0 {
        let cur = zero_in_queue[0];
        zero_in_queue.remove(0);
        ans[cur as usize] += time[cur as usize];
        for next in nexts[cur as usize].iter() {
            ans[*next as usize] = get_max(ans[*next as usize], ans[cur as usize]);
            in0[*next as usize] -= 1;
            if in0[*next as usize] == 0 {
                zero_in_queue.push(*next);
            }
        }
    }
    return ans;
}

fn get_max<T: Clone + Copy + std::cmp::PartialOrd>(a: T, b: T) -> T {
    if a > b {
        a
    } else {
        b
    }
}

执行结果如下：

左神 java 代码

关于python numpy array 与 matrix 乘方和python array和matrix的介绍已经告一段落，感谢您的耐心阅读，如果想了解更多关于2021-04-18：给定一个二维数组 matrix，里面的值不是 1 就是 0，上、下、左、右相邻的 1 认为是一片岛，返回 matrix 中、2021-12-28：给定一个二维数组 matrix，matrix [i][j] = k 代表：从 (i,j) 位置可以随意往右跳＜=k 步，或者从 (i,j) 位置可以随意往下跳＜=k 步， 如果 matrix [i]、2022-06-11：注意本文件中，graph 不是邻接矩阵的含义，而是一个二部图。 在长度为 N 的邻接矩阵 matrix 中，所有的点有 N 个，matrix [i][j] 表示点 i 到点 j 的距离或者权重， 而在二部、2022-06-25：给定一个正数 n, 表示有 0~n-1 号任务， 给定一个长度为 n 的数组 time，time [i] 表示 i 号任务做完的时间， 给定一个二维数组 matrix， matrix [j] = {a,的相关信息，请在本站寻找。